Products You May Like
A group of mathematicians has unveiled a brand new 13-sided shape they’ve simply called ‘the hat’.
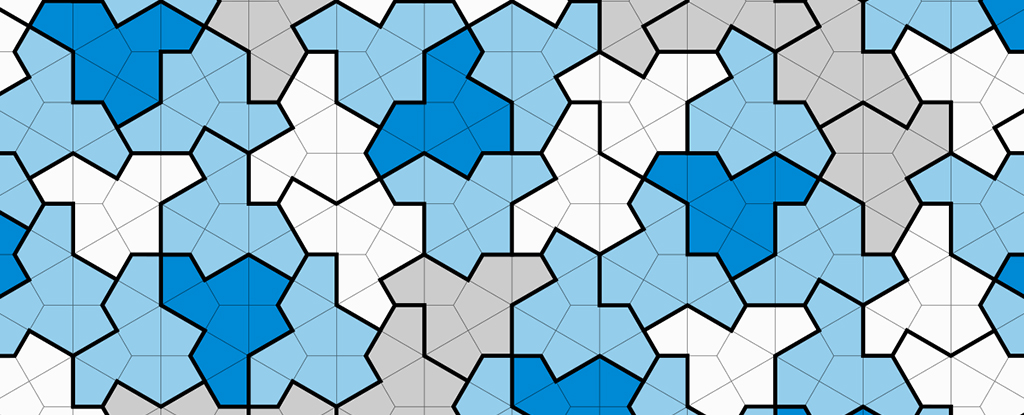
Don’t let the rather mundane description fool you though. This hat (which looks a bit like a fedora) is next season’s must-have fashion item, able to be tiled across a plane to create patterns that never repeat.
Shapes like these are known as aperiodic monotiles, or einsteins. Slotted together, it’s impossible to find a matching arrangement or orientation somewhere directly above or on the same horizon.
The hat was first identified by non-professional mathematician and “shape hobbyist” David Smith from the UK. He did some tinkering in a shape generation program before moving to physical paper cut-outs.
Enlisting the help of academics from the University of Waterloo in Canada and the University of Arkansas, Smith was able to prove the shape was indeed an aperiodic monotile through the use of computer algorithms.
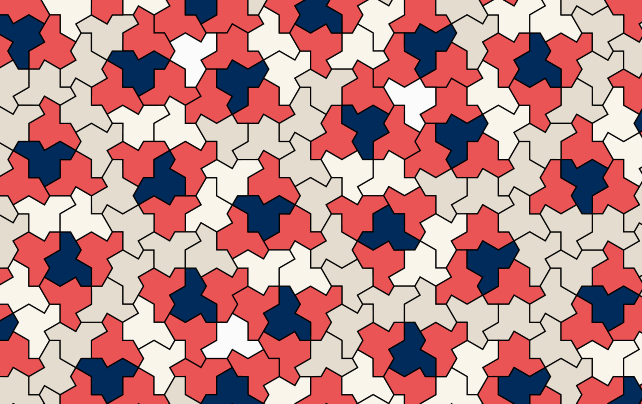
“Aperiodic sets of tiles walk a fine line between order and disorder, admitting tilings, but only those without any translational symmetry, never permitting the simple repetition of periodic tiling,” write the members of the team in their paper.
The very first aperiodic set of tiles was discovered in 1966 and consisted of 20,426 shapes. Over the years, that number has come down, and there are now several aperiodic sets of tiles made up of just two shapes.
Before now though, no one has come up with a single tile that fits the criteria. It’s something that many mathematicians have been looking for since the 1960s, which gives you an idea of the importance of this discovery.
The shape is also a polykite, the name given to shapes made up of multiples of a quadrilateral kite shape.
According to the people who identified the hat as an aperiodic monotile, more discoveries along similar lines could be on the way – more einsteins (not named after the physicist, but rather German for “one stone”) might be out there waiting to be found.
“Several candidate tiles have been proposed as einsteins, but they all challenge in some way the concepts of ’tile’, ’tiling’, or ‘aperiodic’,” write the researchers.
When you take a look at the hat, it seems simple enough that it would have been likely to have been found several decades ago – and indeed the researchers themselves call it “almost mundane in its simplicity”.
The team has also introduced a new method for proving the existence of future einsteins, where various permutations of the shape are combined in order to help establish that they can continue on forever without becoming symmetrical in their patterns.
It remains to be seen how the hat is going to be used by researchers, mathematicians and artists in the future – but it opens up all kinds of avenues to explore, not least whether or not there is a finite number of aperiodic monotiles out there, waiting to be found.
“Finding such a monotile pushes the boundaries of complexity known to be achievable by the tiling behaviour of a single closed topological disk,” write the researchers.
The paper describing the new hat shape has yet to be peer reviewed but can be accessed online at arXiv.